Concurrency of bisectors

Theorem 3

Prove that: The right bisectors of the sides of a triangle are concurrent.
Given: A triangle $ABC$
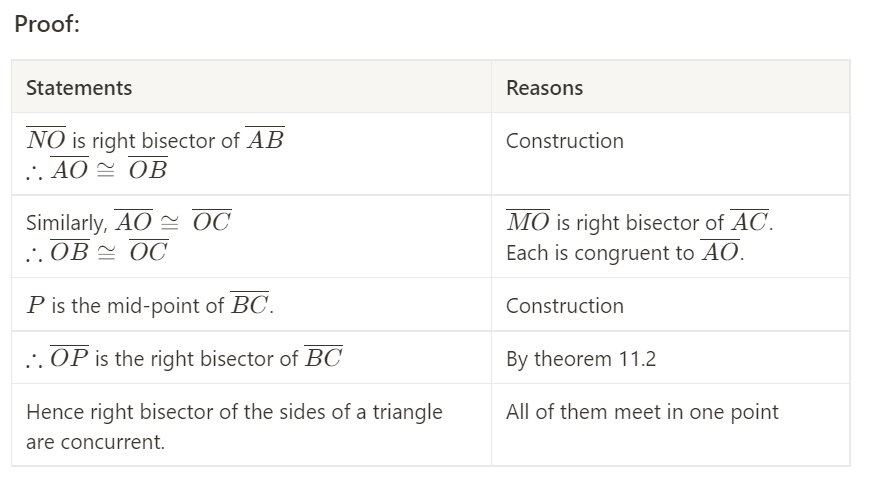
To Prove: The right bisectors of the sides are concurrent.

Construction: Draw $\overline{NO},\overline{MO},$ the right bisectors of $\overline{AB}$ and $\overline{AC}$ meeting in $O$. Bisect $\overline{BC}$ at $P$. Draw $\overline{OP},\overline{OA}, \overline{OB}, \overline{OC}.$

• Explanation

  • To prove that the right bisectors of the sides of a triangle are concurrent, we need to show that they all meet at a single point. Let's call this point O. To do this, we draw the right bisectors of $\overline{AB}$ i.e $\overline{NO}$ and $\overline{AC}$ i.e $\overline{MO}$ and let them meet at point $O$. Then we bisect $\overline{BC}$ at point $P$ and draw $\overline{OP}, \overline{OA}, \overline{OB},$ and $\overline{OC}$.

  • Now, we need to show that all of these lines are congruent, which will prove that they all meet at point $O$. We know that $\overline{NO}$ is the right bisector of $\overline{AB}$ and $\overline{MO}$ is the right bisector of $\overline{AC}$, so they are both perpendicular to their respective sides and divide them into two equal parts. This means that $\overline{AO}$ is congruent to $\overline{OB}$ and also to $\overline{OC}$. Therefore, $\overline{OB}$ is congruent to $\overline{OC}$, which means that $O$ lies on the perpendicular bisector of $\overline{BC}$ as well.

  • Since $\overline{OP}$ is also the perpendicular bisector of $\overline{BC}$, we have shown that all three lines - $\overline{NO}$, $\overline{MO}$, and $\overline{OP}$ - meet at point $O$. Therefore, the right bisectors of the sides of a triangle are concurrent.

Theorem 4

Prove that: Any point on the bisector of an angle is equidistant from its arms.
Given: $\overrightarrow{BD}$ is the bisector of $\angle ABC$. $P$ is any point on $\overrightarrow{BD}$. $\overline{PQ}$ and $\overline{PQ}$ are perpendicular on $\overrightarrow{BA }$ and $\overrightarrow{BC}$ respectively.

To Prove: $\overline{PQ} \cong \overline{PR}$ (i.e. point $P$ is equidistant from $BA$ and $BC$)

• Explanation

  • The statement to be proved in this proof is that any point on the bisector of an angle is equidistant from its arms. To prove this, the given diagram shows a point $P$ on the bisector $\overrightarrow{BD}$ of angle $\angle ABC$. Lines $\overline{PQ}$ and $\overline{PR}$ are drawn perpendicular to sides $\overline{BA}$ and $\overline{BC}$, respectively.

  • To begin the proof, we first look at triangles $\triangle PQB$ and $\triangle PRB$. These triangles share a common side $\overline{PB}$, and angles $\angle PQB$ and $\angle PRB$ are both right angles since $\overline{PQ}$ and $\overline{PR}$ are perpendicular to the sides of the triangle. Additionally, since point $P$ lies on the bisector of angle $\angle ABC$, we know that angles $\angle PBC$ and $\angle ABP$ are congruent. This means that angles $\angle 3$ and $\angle 4$ in triangles $\triangle PQB$ and $\triangle PRB$ are also congruent.

  • Next, we look at angles $\angle 1$ and $\angle 2$ in these triangles. Since $\overline{PQ}$ and $\overline{PR}$ are both perpendicular to sides of the triangle, we know that $\angle 1$ and $\angle 2$ are also congruent right angles. Finally, we see that $\overline{BP}$ is common to both triangles and is congruent to itself.

  • Using the Angle-Angle-Side (AAS) congruence criterion, we can conclude that $\triangle PQB$ is congruent to $\triangle PRB$. Therefore, the corresponding sides of these triangles are congruent, which means that $\overline{PQ}$ is congruent to $\overline{PR}$. This tells us that point $P$ is equidistant from the sides $\overrightarrow{BA}$ and $\overrightarrow{BC}$ of angle $\angle ABC$. Thus, the statement to be proved has been shown to be true.

Theorem 5

Prove that: Any point inside an angle, equidistant from its arms, is on the bisector of it. (Converse of Theorem 11.4)
Given: $P$ is any point on $\overrightarrow{BD}$ equidistant from the arms $\overrightarrow{BA }$ and $\overrightarrow{BC}$ of $\angle ABC$, i.e. $\overline{PQ} \cong \overline{PR}$ and $\overline{PQ} \perp \overline{BA}$ and $\overline{PR} \perp \overline{BC}$

To Prove: $\angle 1 \cong \angle 2$ i.e. $BD$ is the bisector of $\angle ABC$.

• Explanation

  • The theorem states that if a point is located inside an angle and is equidistant from the two arms of the angle, then it must lie on the bisector of that angle.

  • Let's imagine that we have an angle, say angle $ABC$, and there is a point $P$ inside it such that $\overline{PQ}$ and $\overline{PR}$ are perpendicular to the two arms of the angle, and $\overline{PQ} \cong \overline{PR}$. We need to prove that the line $\overrightarrow{BD}$ that bisects the angle must pass through the point $P$.

  • To prove this, we draw two right triangles, $\triangle PQB$ and $\triangle PRB$, which share a common hypotenuse, $\overline{BP}$. By using the fact that the triangles are right-angled, we can show that $\angle 1$ is congruent to $\angle 2$, and therefore, the line $\overrightarrow{BD}$ bisects the angle $ABC$.

  So, in simple terms, if a point is at an equal distance from the two arms of an angle, it will lie on the bisector of that angle.

Theorem 6

Prove that: The bisectors of the angle of a triangle are concurrent
Given: In $\triangle ABC$, $\overline {BE}$ and $\overline {CF}$ are the bisectors of $\angle B$ and $\angle C$ respectively which intersect each other at point $O$.
To Prove: The bisectors of $\angle A$, $\angle B$ and $\angle C$ are concurrent.

Construction: Draw $\overline{OF} \perp \overline{AB}$ and $\overline{OD} \perp \overline{BC}$

Explanation

• In this proof, we are going to show that the bisectors of the angles of a triangle are concurrent.

• We are given a triangle $\triangle ABC$, in which $\overline {BE}$ and $\overline {CF}$ are the bisectors of angles $\angle B$ and $\angle C$ respectively, and they intersect each other at point $O$. Our goal is to prove that the bisectors of angles $\angle A$, $\angle B$, and $\angle C$ are concurrent.

• To begin with, we construct $\overline{OF} \perp \overline{AB}$ and $\overline{OD} \perp \overline{BC}$, as shown in the figure.

• Now, we observe that the point $O$ lies on both $\overline{OD}$ and $\overline{OF}$, and since $OD$ and $OF$ are perpendicular bisectors of $\overline{BC}$and $\overline{AB}$ respectively, we have $\overline{OD} \cong \overline{OF}$ and $\overline{OD} \cong \overline{OE}$.

• So, we conclude that $O$ is equidistant from $\overline{AB} ,\overline{BC}$, and $\overline{CA}$, and hence lies on the bisector of angle $\angle A$. Also, $O$ is already on the bisector of $\angle B$ and $\angle C$.

• Therefore, we have shown that the bisectors of angles $\angle A,\angle B$, and $\angle C$ are concurrent at point $O$, which completes the proof.